Geant4  10.03.p01
 All Classes Namespaces Files Functions Variables Typedefs Enumerations Enumerator Friends Macros Pages
Public Types | Public Member Functions | List of all members
G4Trd Class Reference

#include <G4Trd.hh>

Inheritance diagram for G4Trd:
Inheritance graph
[legend]
Collaboration diagram for G4Trd:
Collaboration graph
[legend]

Public Types

enum  ESide {
  kUndefined, kPX, kMX, kPY,
  kMY, kPZ, kMZ
}
 

Public Member Functions

 G4Trd (const G4String &pName, G4double pdx1, G4double pdx2, G4double pdy1, G4double pdy2, G4double pdz)
 
 ~G4Trd ()
 
G4double GetXHalfLength1 () const
 
G4double GetXHalfLength2 () const
 
G4double GetYHalfLength1 () const
 
G4double GetYHalfLength2 () const
 
G4double GetZHalfLength () const
 
void SetXHalfLength1 (G4double val)
 
void SetXHalfLength2 (G4double val)
 
void SetYHalfLength1 (G4double val)
 
void SetYHalfLength2 (G4double val)
 
void SetZHalfLength (G4double val)
 
G4double GetCubicVolume ()
 
G4double GetSurfaceArea ()
 
void ComputeDimensions (G4VPVParameterisation *p, const G4int n, const G4VPhysicalVolume *pRep)
 
void Extent (G4ThreeVector &pMin, G4ThreeVector &pMax) const
 
G4bool CalculateExtent (const EAxis pAxis, const G4VoxelLimits &pVoxelLimit, const G4AffineTransform &pTransform, G4double &pMin, G4double &pMax) const
 
EInside Inside (const G4ThreeVector &p) const
 
G4ThreeVector SurfaceNormal (const G4ThreeVector &p) const
 
G4double DistanceToIn (const G4ThreeVector &p, const G4ThreeVector &v) const
 
G4double DistanceToIn (const G4ThreeVector &p) const
 
G4double DistanceToOut (const G4ThreeVector &p, const G4ThreeVector &v, const G4bool calcNorm=false, G4bool *validNorm=0, G4ThreeVector *n=0) const
 
G4double DistanceToOut (const G4ThreeVector &p) const
 
void CheckAndSetAllParameters (G4double pdx1, G4double pdx2, G4double pdy1, G4double pdy2, G4double pdz)
 
void SetAllParameters (G4double pdx1, G4double pdx2, G4double pdy1, G4double pdy2, G4double pdz)
 
G4GeometryType GetEntityType () const
 
G4ThreeVector GetPointOnSurface () const
 
G4VSolid * Clone () const
 
std::ostream & StreamInfo (std::ostream &os) const
 
void DescribeYourselfTo (G4VGraphicsScene &scene) const
 
G4Polyhedron * CreatePolyhedron () const
 
 G4Trd (__void__ &)
 
 G4Trd (const G4Trd &rhs)
 
G4Trd & operator= (const G4Trd &rhs)
 
G4ThreeVector ApproxSurfaceNormal (const G4ThreeVector &p) const
 
- Public Member Functions inherited from G4CSGSolid
 G4CSGSolid (const G4String &pName)
 
virtual ~G4CSGSolid ()
 
virtual G4Polyhedron * GetPolyhedron () const
 
 G4CSGSolid (__void__ &)
 
 G4CSGSolid (const G4CSGSolid &rhs)
 
G4CSGSolid & operator= (const G4CSGSolid &rhs)
 
- Public Member Functions inherited from G4VSolid
 G4VSolid (const G4String &name)
 
virtual ~G4VSolid ()
 
G4bool operator== (const G4VSolid &s) const
 
G4String GetName () const
 
void SetName (const G4String &name)
 
G4double GetTolerance () const
 
void DumpInfo () const
 
virtual G4VisExtent GetExtent () const
 
virtual const G4VSolid * GetConstituentSolid (G4int no) const
 
virtual G4VSolid * GetConstituentSolid (G4int no)
 
virtual const G4DisplacedSolid * GetDisplacedSolidPtr () const
 
virtual G4DisplacedSolid * GetDisplacedSolidPtr ()
 
 G4VSolid (__void__ &)
 
 G4VSolid (const G4VSolid &rhs)
 
G4VSolid & operator= (const G4VSolid &rhs)
 
G4double EstimateCubicVolume (G4int nStat, G4double epsilon) const
 
G4double EstimateSurfaceArea (G4int nStat, G4double ell) const
 

Additional Inherited Members

- Protected Member Functions inherited from G4CSGSolid
G4double GetRadiusInRing (G4double rmin, G4double rmax) const
 
- Protected Member Functions inherited from G4VSolid
void CalculateClippedPolygonExtent (G4ThreeVectorList &pPolygon, const G4VoxelLimits &pVoxelLimit, const EAxis pAxis, G4double &pMin, G4double &pMax) const
 
void ClipCrossSection (G4ThreeVectorList *pVertices, const G4int pSectionIndex, const G4VoxelLimits &pVoxelLimit, const EAxis pAxis, G4double &pMin, G4double &pMax) const
 
void ClipBetweenSections (G4ThreeVectorList *pVertices, const G4int pSectionIndex, const G4VoxelLimits &pVoxelLimit, const EAxis pAxis, G4double &pMin, G4double &pMax) const
 
void ClipPolygon (G4ThreeVectorList &pPolygon, const G4VoxelLimits &pVoxelLimit, const EAxis pAxis) const
 
- Protected Attributes inherited from G4CSGSolid
G4double fCubicVolume
 
G4double fSurfaceArea
 
G4bool fRebuildPolyhedron
 
G4Polyhedron * fpPolyhedron
 
- Protected Attributes inherited from G4VSolid
G4double kCarTolerance
 

Detailed Description

Definition at line 72 of file G4Trd.hh.

Member Enumeration Documentation

enum G4Trd::ESide
Enumerator
kUndefined 
kPX 
kMX 
kPY 
kMY 
kPZ 
kMZ 

Definition at line 159 of file G4Trd.hh.

159 {kUndefined, kPX,kMX,kPY,kMY,kPZ,kMZ};

Constructor & Destructor Documentation

G4Trd::G4Trd ( const G4String &  pName,
G4double  pdx1,
G4double  pdx2,
G4double  pdy1,
G4double  pdy2,
G4double  pdz 
)

Definition at line 64 of file G4Trd.cc.

68  : G4CSGSolid(pName)
69 {
70  CheckAndSetAllParameters (pdx1, pdx2, pdy1, pdy2, pdz);
71 }
G4CSGSolid::G4CSGSolid
G4CSGSolid(const G4String &pName)
Definition: G4CSGSolid.cc:49
G4Trd::CheckAndSetAllParameters
void CheckAndSetAllParameters(G4double pdx1, G4double pdx2, G4double pdy1, G4double pdy2, G4double pdz)
Definition: G4Trd.cc:77

Here is the call graph for this function:

Here is the caller graph for this function:

G4Trd::~G4Trd ( )

Definition at line 132 of file G4Trd.cc.

133 {
134 }
G4Trd::G4Trd ( __void__ &  a)

Definition at line 123 of file G4Trd.cc.

124  : G4CSGSolid(a), fDx1(0.), fDx2(0.), fDy1(0.), fDy2(0.), fDz(0.)
125 {
126 }
G4CSGSolid::G4CSGSolid
G4CSGSolid(const G4String &pName)
Definition: G4CSGSolid.cc:49
G4Trd::G4Trd ( const G4Trd &  rhs)

Definition at line 140 of file G4Trd.cc.

141  : G4CSGSolid(rhs), fDx1(rhs.fDx1), fDx2(rhs.fDx2),
142  fDy1(rhs.fDy1), fDy2(rhs.fDy2), fDz(rhs.fDz)
143 {
144 }
G4CSGSolid::G4CSGSolid
G4CSGSolid(const G4String &pName)
Definition: G4CSGSolid.cc:49

Member Function Documentation

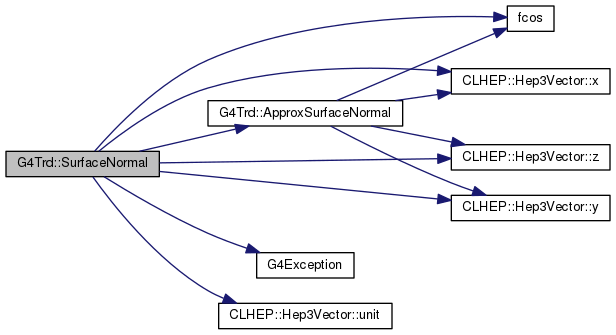
G4ThreeVector G4Trd::ApproxSurfaceNormal ( const G4ThreeVector &  p) const

Definition at line 410 of file G4Trd.cc.

411 {
412  G4ThreeVector norm;
413  G4double z,tanx,secx,newpx,widx;
414  G4double tany,secy,newpy,widy;
415  G4double distx,disty,distz,fcos;
416 
417  z=2.0*fDz;
418 
419  tanx=(fDx2-fDx1)/z;
420  secx=std::sqrt(1.0+tanx*tanx);
421  newpx=std::fabs(p.x())-p.z()*tanx;
422  widx=fDx2-fDz*tanx;
423 
424  tany=(fDy2-fDy1)/z;
425  secy=std::sqrt(1.0+tany*tany);
426  newpy=std::fabs(p.y())-p.z()*tany;
427  widy=fDy2-fDz*tany;
428 
429  distx=std::fabs(newpx-widx)/secx; // perpendicular distance to x side
430  disty=std::fabs(newpy-widy)/secy; // to y side
431  distz=std::fabs(std::fabs(p.z())-fDz); // to z side
432 
433  // find closest side
434  //
435  if (distx<=disty)
436  {
437  if (distx<=distz)
438  {
439  // Closest to X
440  //
441  fcos=1.0/secx;
442  // normal=(+/-std::cos(ang),0,-std::sin(ang))
443  if (p.x()>=0)
444  norm=G4ThreeVector(fcos,0,-tanx*fcos);
445  else
446  norm=G4ThreeVector(-fcos,0,-tanx*fcos);
447  }
448  else
449  {
450  // Closest to Z
451  //
452  if (p.z()>=0)
453  norm=G4ThreeVector(0,0,1);
454  else
455  norm=G4ThreeVector(0,0,-1);
456  }
457  }
458  else
459  {
460  if (disty<=distz)
461  {
462  // Closest to Y
463  //
464  fcos=1.0/secy;
465  if (p.y()>=0)
466  norm=G4ThreeVector(0,fcos,-tany*fcos);
467  else
468  norm=G4ThreeVector(0,-fcos,-tany*fcos);
469  }
470  else
471  {
472  // Closest to Z
473  //
474  if (p.z()>=0)
475  norm=G4ThreeVector(0,0,1);
476  else
477  norm=G4ThreeVector(0,0,-1);
478  }
479  }
480  return norm;
481 }
G4ThreeVector
CLHEP::Hep3Vector G4ThreeVector
Definition: G4ThreeVector.hh:42
CLHEP::Hep3Vector::x
double x() const
fcos
G4double fcos(G4double arg)
Definition: G4tgrEvaluator.cc:68
CLHEP::Hep3Vector::z
double z() const
CLHEP::Hep3Vector::y
double y() const
G4double
double G4double
Definition: G4Types.hh:76

Here is the call graph for this function:

Here is the caller graph for this function:

G4bool G4Trd::CalculateExtent ( const EAxis  pAxis,
const G4VoxelLimits &  pVoxelLimit,
const G4AffineTransform &  pTransform,
G4double &  pMin,
G4double &  pMax 
) const
virtual

Implements G4VSolid.

Definition at line 227 of file G4Trd.cc.

231 {
232  G4ThreeVector bmin, bmax;
233  G4bool exist;
234 
235  // Check bounding box (bbox)
236  //
237  Extent(bmin,bmax);
238  G4BoundingEnvelope bbox(bmin,bmax);
239 #ifdef G4BBOX_EXTENT
240  if (true) return bbox.CalculateExtent(pAxis,pVoxelLimit,pTransform,pMin,pMax);
241 #endif
242  if (bbox.BoundingBoxVsVoxelLimits(pAxis,pVoxelLimit,pTransform,pMin,pMax))
243  {
244  return exist = (pMin < pMax) ? true : false;
245  }
246 
247  // Set bounding envelope (benv) and calculate extent
248  //
249  G4double dx1 = GetXHalfLength1();
250  G4double dx2 = GetXHalfLength2();
251  G4double dy1 = GetYHalfLength1();
252  G4double dy2 = GetYHalfLength2();
253  G4double dz = GetZHalfLength();
254 
255  G4ThreeVectorList baseA(4), baseB(4);
256  baseA[0].set(-dx1,-dy1,-dz);
257  baseA[1].set( dx1,-dy1,-dz);
258  baseA[2].set( dx1, dy1,-dz);
259  baseA[3].set(-dx1, dy1,-dz);
260  baseB[0].set(-dx2,-dy2, dz);
261  baseB[1].set( dx2,-dy2, dz);
262  baseB[2].set( dx2, dy2, dz);
263  baseB[3].set(-dx2, dy2, dz);
264 
265  std::vector<const G4ThreeVectorList *> polygons(2);
266  polygons[0] = &baseA;
267  polygons[1] = &baseB;
268 
269  G4BoundingEnvelope benv(bmin,bmax,polygons);
270  exist = benv.CalculateExtent(pAxis,pVoxelLimit,pTransform,pMin,pMax);
271  return exist;
272 }
G4Trd::GetYHalfLength1
G4double GetYHalfLength1() const
G4Trd::GetZHalfLength
G4double GetZHalfLength() const
G4Trd::GetXHalfLength2
G4double GetXHalfLength2() const
G4Trd::Extent
void Extent(G4ThreeVector &pMin, G4ThreeVector &pMax) const
Definition: G4Trd.cc:196
G4bool
bool G4bool
Definition: G4Types.hh:79
G4Trd::GetYHalfLength2
G4double GetYHalfLength2() const
G4ThreeVectorList
std::vector< G4ThreeVector > G4ThreeVectorList
Definition: G4BoundingEnvelope.hh:61
G4Trd::GetXHalfLength1
G4double GetXHalfLength1() const
G4double
double G4double
Definition: G4Types.hh:76

Here is the call graph for this function:

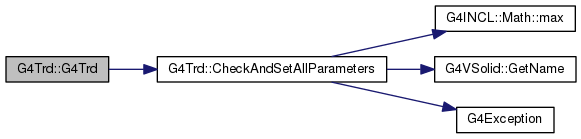
void G4Trd::CheckAndSetAllParameters ( G4double  pdx1,
G4double  pdx2,
G4double  pdy1,
G4double  pdy2,
G4double  pdz 
)

Definition at line 77 of file G4Trd.cc.

80 {
81  if ( pdx1>0&&pdx2>0&&pdy1>0&&pdy2>0&&pdz>0 )
82  {
83  fDx1=pdx1; fDx2=pdx2;
84  fDy1=pdy1; fDy2=pdy2;
85  fDz=pdz;
86  }
87  else
88  {
89  if ( pdx1>=0 && pdx2>=0 && pdy1>=0 && pdy2>=0 && pdz>=0 )
90  {
91  // G4double Minimum_length= (1+per_thousand) * kCarTolerance/2.;
92  // FIX-ME : temporary solution for ZERO or very-small parameters
93  //
94  G4double Minimum_length= kCarTolerance/2.;
95  fDx1=std::max(pdx1,Minimum_length);
96  fDx2=std::max(pdx2,Minimum_length);
97  fDy1=std::max(pdy1,Minimum_length);
98  fDy2=std::max(pdy2,Minimum_length);
99  fDz=std::max(pdz,Minimum_length);
100  }
101  else
102  {
103  std::ostringstream message;
104  message << "Invalid negative dimensions for Solid: " << GetName()
105  << G4endl
106  << " X - " << pdx1 << ", " << pdx2 << G4endl
107  << " Y - " << pdy1 << ", " << pdy2 << G4endl
108  << " Z - " << pdz;
109  G4Exception("G4Trd::CheckAndSetAllParameters()",
110  "GeomSolids0002", FatalException, message);
111  }
112  }
113  fCubicVolume= 0.;
114  fSurfaceArea= 0.;
115  fRebuildPolyhedron = true;
116 }
G4VSolid::GetName
G4String GetName() const
G4CSGSolid::fRebuildPolyhedron
G4bool fRebuildPolyhedron
Definition: G4CSGSolid.hh:80
G4CSGSolid::fCubicVolume
G4double fCubicVolume
Definition: G4CSGSolid.hh:78
G4CSGSolid::fSurfaceArea
G4double fSurfaceArea
Definition: G4CSGSolid.hh:79
G4Exception
void G4Exception(const char *originOfException, const char *exceptionCode, G4ExceptionSeverity severity, const char *comments)
Definition: G4Exception.cc:41
G4INCL::Math::max
T max(const T t1, const T t2)
brief Return the largest of the two arguments
Definition: G4INCLGlobals.hh:112
FatalException
Definition: G4ExceptionSeverity.hh:60
G4endl
#define G4endl
Definition: G4ios.hh:61
G4VSolid::kCarTolerance
G4double kCarTolerance
Definition: G4VSolid.hh:307
G4double
double G4double
Definition: G4Types.hh:76

Here is the call graph for this function:

Here is the caller graph for this function:

G4VSolid * G4Trd::Clone ( ) const
virtual

Reimplemented from G4VSolid.

Definition at line 1229 of file G4Trd.cc.

1230 {
1231  return new G4Trd(*this);
1232 }
G4Trd::G4Trd
G4Trd(const G4String &pName, G4double pdx1, G4double pdx2, G4double pdy1, G4double pdy2, G4double pdz)
Definition: G4Trd.cc:64

Here is the call graph for this function:

void G4Trd::ComputeDimensions ( G4VPVParameterisation *  p,
const G4int  n,
const G4VPhysicalVolume *  pRep 
)
virtual

Reimplemented from G4VSolid.

Definition at line 185 of file G4Trd.cc.

188 {
189  p->ComputeDimensions(*this,n,pRep);
190 }
G4VPVParameterisation::ComputeDimensions
virtual void ComputeDimensions(G4Box &, const G4int, const G4VPhysicalVolume *) const
Definition: G4VPVParameterisation.hh:95

Here is the call graph for this function:

G4Polyhedron * G4Trd::CreatePolyhedron ( ) const
virtual

Reimplemented from G4VSolid.

Definition at line 1333 of file G4Trd.cc.

1334 {
1335  return new G4PolyhedronTrd2 (fDx1, fDx2, fDy1, fDy2, fDz);
1336 }
void G4Trd::DescribeYourselfTo ( G4VGraphicsScene &  scene) const
virtual

Implements G4VSolid.

Definition at line 1328 of file G4Trd.cc.

1329 {
1330  scene.AddSolid (*this);
1331 }
G4VGraphicsScene::AddSolid
virtual void AddSolid(const G4Box &)=0

Here is the call graph for this function:

G4double G4Trd::DistanceToIn ( const G4ThreeVector &  p,
const G4ThreeVector &  v 
) const
virtual

Implements G4VSolid.

Definition at line 503 of file G4Trd.cc.

505 {
506  G4double snxt = kInfinity ; // snxt = default return value
507  G4double smin,smax;
508  G4double s1,s2,tanxz,tanyz,ds1,ds2;
509  G4double ss1,ss2,sn1=0.,sn2=0.,Dist;
510 
511  if ( v.z() ) // Calculate valid z intersect range
512  {
513  if ( v.z() > 0 ) // Calculate smax: must be +ve or no intersection.
514  {
515  Dist = fDz - p.z() ; // to plane at +dz
516 
517  if (Dist >= 0.5*kCarTolerance)
518  {
519  smax = Dist/v.z() ;
520  smin = -(fDz + p.z())/v.z() ;
521  }
522  else return snxt ;
523  }
524  else // v.z <0
525  {
526  Dist=fDz+p.z(); // plane at -dz
527 
528  if ( Dist >= 0.5*kCarTolerance )
529  {
530  smax = -Dist/v.z() ;
531  smin = (fDz - p.z())/v.z() ;
532  }
533  else return snxt ;
534  }
535  if (smin < 0 ) smin = 0 ;
536  }
537  else // v.z=0
538  {
539  if (std::fabs(p.z()) >= fDz ) return snxt ; // Outside & no intersect
540  else
541  {
542  smin = 0 ; // Always inside z range
543  smax = kInfinity;
544  }
545  }
546 
547  // Calculate x intersection range
548  //
549  // Calc half width at p.z, and components towards planes
550 
551  tanxz = (fDx2 - fDx1)*0.5/fDz ;
552  s1 = 0.5*(fDx1+fDx2) + tanxz*p.z() ; // x half width at p.z
553  ds1 = v.x() - tanxz*v.z() ; // Components of v towards faces at +-x
554  ds2 = v.x() + tanxz*v.z() ;
555  ss1 = s1 - p.x() ; // -delta x to +ve plane
556  // -ve when outside
557  ss2 = -s1 - p.x() ; // -delta x to -ve plane
558  // +ve when outside
559 
560  if (ss1 < 0 && ss2 <= 0 )
561  {
562  if (ds1 < 0) // In +ve coord Area
563  {
564  sn1 = ss1/ds1 ;
565 
566  if ( ds2 < 0 ) sn2 = ss2/ds2 ;
567  else sn2 = kInfinity ;
568  }
569  else return snxt ;
570  }
571  else if ( ss1 >= 0 && ss2 > 0 )
572  {
573  if ( ds2 > 0 ) // In -ve coord Area
574  {
575  sn1 = ss2/ds2 ;
576 
577  if (ds1 > 0) sn2 = ss1/ds1 ;
578  else sn2 = kInfinity;
579 
580  }
581  else return snxt ;
582  }
583  else if (ss1 >= 0 && ss2 <= 0 )
584  {
585  // Inside Area - calculate leaving distance
586  // *Don't* use exact distance to side for tolerance
587  // = ss1*std::cos(ang xz)
588  // = ss1/std::sqrt(1.0+tanxz*tanxz)
589  sn1 = 0 ;
590 
591  if ( ds1 > 0 )
592  {
593  if (ss1 > 0.5*kCarTolerance) sn2 = ss1/ds1 ; // Leave +ve side extent
594  else return snxt ; // Leave immediately by +ve
595  }
596  else sn2 = kInfinity ;
597 
598  if ( ds2 < 0 )
599  {
600  if ( ss2 < -0.5*kCarTolerance )
601  {
602  Dist = ss2/ds2 ; // Leave -ve side extent
603  if ( Dist < sn2 ) sn2 = Dist ;
604  }
605  else return snxt ;
606  }
607  }
608  else if (ss1 < 0 && ss2 > 0 )
609  {
610  // Within +/- plane cross-over areas (not on boundaries ss1||ss2==0)
611 
612  if ( ds1 >= 0 || ds2 <= 0 )
613  {
614  return snxt ;
615  }
616  else // Will intersect & stay inside
617  {
618  sn1 = ss1/ds1 ;
619  Dist = ss2/ds2 ;
620  if (Dist > sn1 ) sn1 = Dist ;
621  sn2 = kInfinity ;
622  }
623  }
624 
625  // Reduce allowed range of distances as appropriate
626 
627  if ( sn1 > smin ) smin = sn1 ;
628  if ( sn2 < smax ) smax = sn2 ;
629 
630  // Check for incompatible ranges (eg z intersects between 50 ->100 and x
631  // only 10-40 -> no intersection)
632 
633  if ( smax < smin ) return snxt ;
634 
635  // Calculate valid y intersection range
636  // (repeat of x intersection code)
637 
638  tanyz = (fDy2-fDy1)*0.5/fDz ;
639  s2 = 0.5*(fDy1+fDy2) + tanyz*p.z() ; // y half width at p.z
640  ds1 = v.y() - tanyz*v.z() ; // Components of v towards faces at +-y
641  ds2 = v.y() + tanyz*v.z() ;
642  ss1 = s2 - p.y() ; // -delta y to +ve plane
643  ss2 = -s2 - p.y() ; // -delta y to -ve plane
644 
645  if ( ss1 < 0 && ss2 <= 0 )
646  {
647  if (ds1 < 0 ) // In +ve coord Area
648  {
649  sn1 = ss1/ds1 ;
650  if ( ds2 < 0 ) sn2 = ss2/ds2 ;
651  else sn2 = kInfinity ;
652  }
653  else return snxt ;
654  }
655  else if ( ss1 >= 0 && ss2 > 0 )
656  {
657  if ( ds2 > 0 ) // In -ve coord Area
658  {
659  sn1 = ss2/ds2 ;
660  if ( ds1 > 0 ) sn2 = ss1/ds1 ;
661  else sn2 = kInfinity ;
662  }
663  else return snxt ;
664  }
665  else if (ss1 >= 0 && ss2 <= 0 )
666  {
667  // Inside Area - calculate leaving distance
668  // *Don't* use exact distance to side for tolerance
669  // = ss1*std::cos(ang yz)
670  // = ss1/std::sqrt(1.0+tanyz*tanyz)
671  sn1 = 0 ;
672 
673  if ( ds1 > 0 )
674  {
675  if (ss1 > 0.5*kCarTolerance) sn2 = ss1/ds1 ; // Leave +ve side extent
676  else return snxt ; // Leave immediately by +ve
677  }
678  else sn2 = kInfinity ;
679 
680  if ( ds2 < 0 )
681  {
682  if ( ss2 < -0.5*kCarTolerance )
683  {
684  Dist = ss2/ds2 ; // Leave -ve side extent
685  if (Dist < sn2) sn2=Dist;
686  }
687  else return snxt ;
688  }
689  }
690  else if (ss1 < 0 && ss2 > 0 )
691  {
692  // Within +/- plane cross-over areas (not on boundaries ss1||ss2==0)
693 
694  if (ds1 >= 0 || ds2 <= 0 )
695  {
696  return snxt ;
697  }
698  else // Will intersect & stay inside
699  {
700  sn1 = ss1/ds1 ;
701  Dist = ss2/ds2 ;
702  if (Dist > sn1 ) sn1 = Dist ;
703  sn2 = kInfinity ;
704  }
705  }
706 
707  // Reduce allowed range of distances as appropriate
708 
709  if ( sn1 > smin) smin = sn1 ;
710  if ( sn2 < smax) smax = sn2 ;
711 
712  // Check for incompatible ranges (eg x intersects between 50 ->100 and y
713  // only 10-40 -> no intersection). Set snxt if ok
714 
715  if ( smax > smin ) snxt = smin ;
716  if (snxt < 0.5*kCarTolerance ) snxt = 0.0 ;
717 
718  return snxt ;
719 }
kInfinity
static const G4double kInfinity
Definition: geomdefs.hh:42
CLHEP::Hep3Vector::x
double x() const
CLHEP::Hep3Vector::z
double z() const
CLHEP::Hep3Vector::y
double y() const
G4VSolid::kCarTolerance
G4double kCarTolerance
Definition: G4VSolid.hh:307
G4double
double G4double
Definition: G4Types.hh:76

Here is the call graph for this function:

G4double G4Trd::DistanceToIn ( const G4ThreeVector &  p) const
virtual

Implements G4VSolid.

Definition at line 729 of file G4Trd.cc.

730 {
731  G4double safe=0.0;
732  G4double tanxz,distx,safx;
733  G4double tanyz,disty,safy;
734  G4double zbase;
735 
736  safe=std::fabs(p.z())-fDz;
737  if (safe<0) safe=0; // Also used to ensure x/y distances
738  // POSITIVE
739 
740  zbase=fDz+p.z();
741 
742  // Find distance along x direction to closest x plane
743  //
744  tanxz=(fDx2-fDx1)*0.5/fDz;
745  // widx=fDx1+tanxz*(fDz+p.z()); // x width at p.z
746  // distx=std::fabs(p.x())-widx; // distance to plane
747  distx=std::fabs(p.x())-(fDx1+tanxz*zbase);
748  if (distx>safe)
749  {
750  safx=distx/std::sqrt(1.0+tanxz*tanxz); // vector Dist=Dist*std::cos(ang)
751  if (safx>safe) safe=safx;
752  }
753 
754  // Find distance along y direction to slanted wall
755  tanyz=(fDy2-fDy1)*0.5/fDz;
756  // widy=fDy1+tanyz*(fDz+p.z()); // y width at p.z
757  // disty=std::fabs(p.y())-widy; // distance to plane
758  disty=std::fabs(p.y())-(fDy1+tanyz*zbase);
759  if (disty>safe)
760  {
761  safy=disty/std::sqrt(1.0+tanyz*tanyz); // distance along vector
762  if (safy>safe) safe=safy;
763  }
764  return safe;
765 }
CLHEP::Hep3Vector::x
double x() const
CLHEP::Hep3Vector::z
double z() const
CLHEP::Hep3Vector::y
double y() const
G4double
double G4double
Definition: G4Types.hh:76

Here is the call graph for this function:

G4double G4Trd::DistanceToOut ( const G4ThreeVector &  p,
const G4ThreeVector &  v,
const G4bool  calcNorm = false,
G4bool *  validNorm = 0,
G4ThreeVector *  n = 0 
) const
virtual

Implements G4VSolid.

Definition at line 776 of file G4Trd.cc.

781 {
782  ESide side = kUndefined, snside = kUndefined;
783  G4double snxt,pdist;
784  G4double central,ss1,ss2,ds1,ds2,sn=0.,sn2=0.;
785  G4double tanxz=0.,cosxz=0.,tanyz=0.,cosyz=0.;
786 
787  if (calcNorm) *validNorm=true; // All normals are valid
788 
789  // Calculate z plane intersection
790  if (v.z()>0)
791  {
792  pdist=fDz-p.z();
793  if (pdist>kCarTolerance/2)
794  {
795  snxt=pdist/v.z();
796  side=kPZ;
797  }
798  else
799  {
800  if (calcNorm)
801  {
802  *n=G4ThreeVector(0,0,1);
803  }
804  return snxt=0;
805  }
806  }
807  else if (v.z()<0)
808  {
809  pdist=fDz+p.z();
810  if (pdist>kCarTolerance/2)
811  {
812  snxt=-pdist/v.z();
813  side=kMZ;
814  }
815  else
816  {
817  if (calcNorm)
818  {
819  *n=G4ThreeVector(0,0,-1);
820  }
821  return snxt=0;
822  }
823  }
824  else
825  {
826  snxt=kInfinity;
827  }
828 
829  //
830  // Calculate x intersection
831  //
832  tanxz=(fDx2-fDx1)*0.5/fDz;
833  central=0.5*(fDx1+fDx2);
834 
835  // +ve plane (1)
836  //
837  ss1=central+tanxz*p.z()-p.x(); // distance || x axis to plane
838  // (+ve if point inside)
839  ds1=v.x()-tanxz*v.z(); // component towards plane at +x
840  // (-ve if +ve -> -ve direction)
841  // -ve plane (2)
842  //
843  ss2=-tanxz*p.z()-p.x()-central; //distance || x axis to plane
844  // (-ve if point inside)
845  ds2=tanxz*v.z()+v.x(); // component towards plane at -x
846 
847  if (ss1>0&&ss2<0)
848  {
849  // Normal case - entirely inside region
850  if (ds1<=0&&ds2<0)
851  {
852  if (ss2<-kCarTolerance/2)
853  {
854  sn=ss2/ds2; // Leave by -ve side
855  snside=kMX;
856  }
857  else
858  {
859  sn=0; // Leave immediately by -ve side
860  snside=kMX;
861  }
862  }
863  else if (ds1>0&&ds2>=0)
864  {
865  if (ss1>kCarTolerance/2)
866  {
867  sn=ss1/ds1; // Leave by +ve side
868  snside=kPX;
869  }
870  else
871  {
872  sn=0; // Leave immediately by +ve side
873  snside=kPX;
874  }
875  }
876  else if (ds1>0&&ds2<0)
877  {
878  if (ss1>kCarTolerance/2)
879  {
880  // sn=ss1/ds1; // Leave by +ve side
881  if (ss2<-kCarTolerance/2)
882  {
883  sn=ss1/ds1; // Leave by +ve side
884  sn2=ss2/ds2;
885  if (sn2<sn)
886  {
887  sn=sn2;
888  snside=kMX;
889  }
890  else
891  {
892  snside=kPX;
893  }
894  }
895  else
896  {
897  sn=0; // Leave immediately by -ve
898  snside=kMX;
899  }
900  }
901  else
902  {
903  sn=0; // Leave immediately by +ve side
904  snside=kPX;
905  }
906  }
907  else
908  {
909  // Must be || to both
910  //
911  sn=kInfinity; // Don't leave by either side
912  }
913  }
914  else if (ss1<=0&&ss2<0)
915  {
916  // Outside, in +ve Area
917 
918  if (ds1>0)
919  {
920  sn=0; // Away from shape
921  // Left by +ve side
922  snside=kPX;
923  }
924  else
925  {
926  if (ds2<0)
927  {
928  // Ignore +ve plane and use -ve plane intersect
929  //
930  sn=ss2/ds2; // Leave by -ve side
931  snside=kMX;
932  }
933  else
934  {
935  // Must be || to both -> exit determined by other axes
936  //
937  sn=kInfinity; // Don't leave by either side
938  }
939  }
940  }
941  else if (ss1>0&&ss2>=0)
942  {
943  // Outside, in -ve Area
944 
945  if (ds2<0)
946  {
947  sn=0; // away from shape
948  // Left by -ve side
949  snside=kMX;
950  }
951  else
952  {
953  if (ds1>0)
954  {
955  // Ignore +ve plane and use -ve plane intersect
956  //
957  sn=ss1/ds1; // Leave by +ve side
958  snside=kPX;
959  }
960  else
961  {
962  // Must be || to both -> exit determined by other axes
963  //
964  sn=kInfinity; // Don't leave by either side
965  }
966  }
967  }
968 
969  // Update minimum exit distance
970 
971  if (sn<snxt)
972  {
973  snxt=sn;
974  side=snside;
975  }
976  if (snxt>0)
977  {
978  // Calculate y intersection
979 
980  tanyz=(fDy2-fDy1)*0.5/fDz;
981  central=0.5*(fDy1+fDy2);
982 
983  // +ve plane (1)
984  //
985  ss1=central+tanyz*p.z()-p.y(); // distance || y axis to plane
986  // (+ve if point inside)
987  ds1=v.y()-tanyz*v.z(); // component towards +ve plane
988  // (-ve if +ve -> -ve direction)
989  // -ve plane (2)
990  //
991  ss2=-tanyz*p.z()-p.y()-central; // distance || y axis to plane
992  // (-ve if point inside)
993  ds2=tanyz*v.z()+v.y(); // component towards -ve plane
994 
995  if (ss1>0&&ss2<0)
996  {
997  // Normal case - entirely inside region
998 
999  if (ds1<=0&&ds2<0)
1000  {
1001  if (ss2<-kCarTolerance/2)
1002  {
1003  sn=ss2/ds2; // Leave by -ve side
1004  snside=kMY;
1005  }
1006  else
1007  {
1008  sn=0; // Leave immediately by -ve side
1009  snside=kMY;
1010  }
1011  }
1012  else if (ds1>0&&ds2>=0)
1013  {
1014  if (ss1>kCarTolerance/2)
1015  {
1016  sn=ss1/ds1; // Leave by +ve side
1017  snside=kPY;
1018  }
1019  else
1020  {
1021  sn=0; // Leave immediately by +ve side
1022  snside=kPY;
1023  }
1024  }
1025  else if (ds1>0&&ds2<0)
1026  {
1027  if (ss1>kCarTolerance/2)
1028  {
1029  // sn=ss1/ds1; // Leave by +ve side
1030  if (ss2<-kCarTolerance/2)
1031  {
1032  sn=ss1/ds1; // Leave by +ve side
1033  sn2=ss2/ds2;
1034  if (sn2<sn)
1035  {
1036  sn=sn2;
1037  snside=kMY;
1038  }
1039  else
1040  {
1041  snside=kPY;
1042  }
1043  }
1044  else
1045  {
1046  sn=0; // Leave immediately by -ve
1047  snside=kMY;
1048  }
1049  }
1050  else
1051  {
1052  sn=0; // Leave immediately by +ve side
1053  snside=kPY;
1054  }
1055  }
1056  else
1057  {
1058  // Must be || to both
1059  //
1060  sn=kInfinity; // Don't leave by either side
1061  }
1062  }
1063  else if (ss1<=0&&ss2<0)
1064  {
1065  // Outside, in +ve Area
1066 
1067  if (ds1>0)
1068  {
1069  sn=0; // Away from shape
1070  // Left by +ve side
1071  snside=kPY;
1072  }
1073  else
1074  {
1075  if (ds2<0)
1076  {
1077  // Ignore +ve plane and use -ve plane intersect
1078  //
1079  sn=ss2/ds2; // Leave by -ve side
1080  snside=kMY;
1081  }
1082  else
1083  {
1084  // Must be || to both -> exit determined by other axes
1085  //
1086  sn=kInfinity; // Don't leave by either side
1087  }
1088  }
1089  }
1090  else if (ss1>0&&ss2>=0)
1091  {
1092  // Outside, in -ve Area
1093  if (ds2<0)
1094  {
1095  sn=0; // away from shape
1096  // Left by -ve side
1097  snside=kMY;
1098  }
1099  else
1100  {
1101  if (ds1>0)
1102  {
1103  // Ignore +ve plane and use -ve plane intersect
1104  //
1105  sn=ss1/ds1; // Leave by +ve side
1106  snside=kPY;
1107  }
1108  else
1109  {
1110  // Must be || to both -> exit determined by other axes
1111  //
1112  sn=kInfinity; // Don't leave by either side
1113  }
1114  }
1115  }
1116 
1117  // Update minimum exit distance
1118 
1119  if (sn<snxt)
1120  {
1121  snxt=sn;
1122  side=snside;
1123  }
1124  }
1125 
1126  if (calcNorm)
1127  {
1128  switch (side)
1129  {
1130  case kPX:
1131  cosxz=1.0/std::sqrt(1.0+tanxz*tanxz);
1132  *n=G4ThreeVector(cosxz,0,-tanxz*cosxz);
1133  break;
1134  case kMX:
1135  cosxz=-1.0/std::sqrt(1.0+tanxz*tanxz);
1136  *n=G4ThreeVector(cosxz,0,tanxz*cosxz);
1137  break;
1138  case kPY:
1139  cosyz=1.0/std::sqrt(1.0+tanyz*tanyz);
1140  *n=G4ThreeVector(0,cosyz,-tanyz*cosyz);
1141  break;
1142  case kMY:
1143  cosyz=-1.0/std::sqrt(1.0+tanyz*tanyz);
1144  *n=G4ThreeVector(0,cosyz,tanyz*cosyz);
1145  break;
1146  case kPZ:
1147  *n=G4ThreeVector(0,0,1);
1148  break;
1149  case kMZ:
1150  *n=G4ThreeVector(0,0,-1);
1151  break;
1152  default:
1153  DumpInfo();
1154  G4Exception("G4Trd::DistanceToOut(p,v,..)",
1155  "GeomSolids1002", JustWarning,
1156  "Undefined side for valid surface normal to solid.");
1157  break;
1158  }
1159  }
1160  return snxt;
1161 }
kInfinity
static const G4double kInfinity
Definition: geomdefs.hh:42
G4ThreeVector
CLHEP::Hep3Vector G4ThreeVector
Definition: G4ThreeVector.hh:42
CLHEP::Hep3Vector::x
double x() const
JustWarning
Definition: G4ExceptionSeverity.hh:64
CLHEP::Hep3Vector::z
double z() const
G4VSolid::DumpInfo
void DumpInfo() const
G4Exception
void G4Exception(const char *originOfException, const char *exceptionCode, G4ExceptionSeverity severity, const char *comments)
Definition: G4Exception.cc:41
CLHEP::Hep3Vector::y
double y() const
G4VSolid::kCarTolerance
G4double kCarTolerance
Definition: G4VSolid.hh:307
ESide
ESide
Definition: G4Cons.cc:76
G4double
double G4double
Definition: G4Types.hh:76

Here is the call graph for this function:

G4double G4Trd::DistanceToOut ( const G4ThreeVector &  p) const
virtual

Implements G4VSolid.

Definition at line 1168 of file G4Trd.cc.

1169 {
1170  G4double safe=0.0;
1171  G4double tanxz,xdist,saf1;
1172  G4double tanyz,ydist,saf2;
1173  G4double zbase;
1174 
1175 #ifdef G4CSGDEBUG
1176  if( Inside(p) == kOutside )
1177  {
1178  G4int oldprc = G4cout.precision(16) ;
1179  G4cout << G4endl ;
1180  DumpInfo();
1181  G4cout << "Position:" << G4endl << G4endl ;
1182  G4cout << "p.x() = " << p.x()/mm << " mm" << G4endl ;
1183  G4cout << "p.y() = " << p.y()/mm << " mm" << G4endl ;
1184  G4cout << "p.z() = " << p.z()/mm << " mm" << G4endl << G4endl ;
1185  G4cout.precision(oldprc) ;
1186  G4Exception("G4Trd::DistanceToOut(p)", "GeomSolids1002", JustWarning,
1187  "Point p is outside !?" );
1188  }
1189 #endif
1190 
1191  safe=fDz-std::fabs(p.z()); // z perpendicular Dist
1192 
1193  zbase=fDz+p.z();
1194 
1195  // xdist = distance perpendicular to z axis to closest x plane from p
1196  // = (x half width of shape at p.z) - std::fabs(p.x)
1197  //
1198  tanxz=(fDx2-fDx1)*0.5/fDz;
1199  xdist=fDx1+tanxz*zbase-std::fabs(p.x());
1200  saf1=xdist/std::sqrt(1.0+tanxz*tanxz); // x*std::cos(ang_xz) =
1201  // shortest (perpendicular)
1202  // distance to plane
1203  tanyz=(fDy2-fDy1)*0.5/fDz;
1204  ydist=fDy1+tanyz*zbase-std::fabs(p.y());
1205  saf2=ydist/std::sqrt(1.0+tanyz*tanyz);
1206 
1207  // Return minimum x/y/z distance
1208  //
1209  if (safe>saf1) safe=saf1;
1210  if (safe>saf2) safe=saf2;
1211 
1212  if (safe<0) safe=0;
1213  return safe;
1214 }
mm
static constexpr double mm
Definition: G4SIunits.hh:115
CLHEP::Hep3Vector::x
double x() const
JustWarning
Definition: G4ExceptionSeverity.hh:64
G4int
int G4int
Definition: G4Types.hh:78
CLHEP::Hep3Vector::z
double z() const
G4VSolid::DumpInfo
void DumpInfo() const
G4cout
G4GLOB_DLL std::ostream G4cout
G4Trd::Inside
EInside Inside(const G4ThreeVector &p) const
Definition: G4Trd.cc:278
G4Exception
void G4Exception(const char *originOfException, const char *exceptionCode, G4ExceptionSeverity severity, const char *comments)
Definition: G4Exception.cc:41
CLHEP::Hep3Vector::y
double y() const
G4endl
#define G4endl
Definition: G4ios.hh:61
G4double
double G4double
Definition: G4Types.hh:76

Here is the call graph for this function:

void G4Trd::Extent ( G4ThreeVector &  pMin,
G4ThreeVector &  pMax 
) const
virtual

Reimplemented from G4VSolid.

Definition at line 196 of file G4Trd.cc.

197 {
198  G4double dx1 = GetXHalfLength1();
199  G4double dx2 = GetXHalfLength2();
200  G4double dy1 = GetYHalfLength1();
201  G4double dy2 = GetYHalfLength2();
202  G4double dz = GetZHalfLength();
203 
204  G4double xmax = std::max(dx1,dx2);
205  G4double ymax = std::max(dy1,dy2);
206  pMin.set(-xmax,-ymax,-dz);
207  pMax.set( xmax, ymax, dz);
208 
209  // Check correctness of the bounding box
210  //
211  if (pMin.x() >= pMax.x() || pMin.y() >= pMax.y() || pMin.z() >= pMax.z())
212  {
213  std::ostringstream message;
214  message << "Bad bounding box (min >= max) for solid: "
215  << GetName() << " !"
216  << "\npMin = " << pMin
217  << "\npMax = " << pMax;
218  G4Exception("G4Trd::Extent()", "GeomMgt0001", JustWarning, message);
219  DumpInfo();
220  }
221 }
CLHEP::Hep3Vector::set
void set(double x, double y, double z)
G4VSolid::GetName
G4String GetName() const
G4Trd::GetYHalfLength1
G4double GetYHalfLength1() const
CLHEP::Hep3Vector::x
double x() const
G4Trd::GetZHalfLength
G4double GetZHalfLength() const
JustWarning
Definition: G4ExceptionSeverity.hh:64
CLHEP::Hep3Vector::z
double z() const
G4VSolid::DumpInfo
void DumpInfo() const
G4Trd::GetXHalfLength2
G4double GetXHalfLength2() const
G4Trd::GetYHalfLength2
G4double GetYHalfLength2() const
G4Exception
void G4Exception(const char *originOfException, const char *exceptionCode, G4ExceptionSeverity severity, const char *comments)
Definition: G4Exception.cc:41
G4INCL::Math::max
T max(const T t1, const T t2)
brief Return the largest of the two arguments
Definition: G4INCLGlobals.hh:112
CLHEP::Hep3Vector::y
double y() const
G4Trd::GetXHalfLength1
G4double GetXHalfLength1() const
G4double
double G4double
Definition: G4Types.hh:76

Here is the call graph for this function:

Here is the caller graph for this function:

G4double G4Trd::GetCubicVolume ( )
inlinevirtual

Reimplemented from G4VSolid.

G4GeometryType G4Trd::GetEntityType ( ) const
virtual

Implements G4VSolid.

Definition at line 1220 of file G4Trd.cc.

1221 {
1222  return G4String("G4Trd");
1223 }
G4ThreeVector G4Trd::GetPointOnSurface ( ) const
virtual

Reimplemented from G4VSolid.

Definition at line 1265 of file G4Trd.cc.

1266 {
1267  G4double px, py, pz, tgX, tgY, secX, secY, select, sumS, tmp;
1268  G4double Sxy1, Sxy2, Sxy, Sxz, Syz;
1269 
1270  tgX = 0.5*(fDx2-fDx1)/fDz;
1271  secX = std::sqrt(1+tgX*tgX);
1272  tgY = 0.5*(fDy2-fDy1)/fDz;
1273  secY = std::sqrt(1+tgY*tgY);
1274 
1275  // calculate 0.25 of side surfaces, sumS is 0.25 of total surface
1276 
1277  Sxy1 = fDx1*fDy1;
1278  Sxy2 = fDx2*fDy2;
1279  Sxy = Sxy1 + Sxy2;
1280  Sxz = (fDx1 + fDx2)*fDz*secY;
1281  Syz = (fDy1 + fDy2)*fDz*secX;
1282  sumS = Sxy + Sxz + Syz;
1283 
1284  select = sumS*G4UniformRand();
1285 
1286  if( select < Sxy ) // Sxy1 or Sxy2
1287  {
1288  if( select < Sxy1 )
1289  {
1290  pz = -fDz;
1291  px = -fDx1 + 2*fDx1*G4UniformRand();
1292  py = -fDy1 + 2*fDy1*G4UniformRand();
1293  }
1294  else
1295  {
1296  pz = fDz;
1297  px = -fDx2 + 2*fDx2*G4UniformRand();
1298  py = -fDy2 + 2*fDy2*G4UniformRand();
1299  }
1300  }
1301  else if ( ( select - Sxy ) < Sxz ) // Sxz
1302  {
1303  pz = -fDz + 2*fDz*G4UniformRand();
1304  tmp = fDx1 + (pz + fDz)*tgX;
1305  px = -tmp + 2*tmp*G4UniformRand();
1306  tmp = fDy1 + (pz + fDz)*tgY;
1307 
1308  if(G4UniformRand() > 0.5) { py = tmp; }
1309  else { py = -tmp; }
1310  }
1311  else // Syz
1312  {
1313  pz = -fDz + 2*fDz*G4UniformRand();
1314  tmp = fDy1 + (pz + fDz)*tgY;
1315  py = -tmp + 2*tmp*G4UniformRand();
1316  tmp = fDx1 + (pz + fDz)*tgX;
1317 
1318  if(G4UniformRand() > 0.5) { px = tmp; }
1319  else { px = -tmp; }
1320  }
1321  return G4ThreeVector(px,py,pz);
1322 }
G4ThreeVector
CLHEP::Hep3Vector G4ThreeVector
Definition: G4ThreeVector.hh:42
G4UniformRand
#define G4UniformRand()
Definition: Randomize.hh:97
G4double
double G4double
Definition: G4Types.hh:76
G4double G4Trd::GetSurfaceArea ( )
inlinevirtual

Reimplemented from G4VSolid.

G4double G4Trd::GetXHalfLength1 ( ) const
inline

Here is the caller graph for this function:

G4double G4Trd::GetXHalfLength2 ( ) const
inline

Here is the caller graph for this function:

G4double G4Trd::GetYHalfLength1 ( ) const
inline

Here is the caller graph for this function:

G4double G4Trd::GetYHalfLength2 ( ) const
inline

Here is the caller graph for this function:

G4double G4Trd::GetZHalfLength ( ) const
inline

Here is the caller graph for this function:

EInside G4Trd::Inside ( const G4ThreeVector &  p) const
virtual

Implements G4VSolid.

Definition at line 278 of file G4Trd.cc.

279 {
280  EInside in=kOutside;
281  G4double x,y,zbase1,zbase2;
282 
283  if (std::fabs(p.z())<=fDz-kCarTolerance/2)
284  {
285  zbase1=p.z()+fDz; // Dist from -ve z plane
286  zbase2=fDz-p.z(); // Dist from +ve z plane
287 
288  // Check whether inside x tolerance
289  //
290  x=0.5*(fDx2*zbase1+fDx1*zbase2)/fDz - kCarTolerance/2;
291  if (std::fabs(p.x())<=x)
292  {
293  y=0.5*((fDy2*zbase1+fDy1*zbase2))/fDz - kCarTolerance/2;
294  if (std::fabs(p.y())<=y)
295  {
296  in=kInside;
297  }
298  else if (std::fabs(p.y())<=y+kCarTolerance)
299  {
300  in=kSurface;
301  }
302  }
303  else if (std::fabs(p.x())<=x+kCarTolerance)
304  {
305  // y = y half width of shape at z of point + tolerant boundary
306  //
307  y=0.5*((fDy2*zbase1+fDy1*zbase2))/fDz + kCarTolerance/2;
308  if (std::fabs(p.y())<=y)
309  {
310  in=kSurface;
311  }
312  }
313  }
314  else if (std::fabs(p.z())<=fDz+kCarTolerance/2)
315  {
316  // Only need to check outer tolerant boundaries
317  //
318  zbase1=p.z()+fDz; // Dist from -ve z plane
319  zbase2=fDz-p.z(); // Dist from +ve z plane
320 
321  // x = x half width of shape at z of point plus tolerance
322  //
323  x=0.5*(fDx2*zbase1+fDx1*zbase2)/fDz + kCarTolerance/2;
324  if (std::fabs(p.x())<=x)
325  {
326  // y = y half width of shape at z of point
327  //
328  y=0.5*((fDy2*zbase1+fDy1*zbase2))/fDz + kCarTolerance/2;
329  if (std::fabs(p.y())<=y) in=kSurface;
330  }
331  }
332  return in;
333 }
CLHEP::Hep3Vector::x
double x() const
CLHEP::Hep3Vector::z
double z() const
EInside
EInside
Definition: geomdefs.hh:58
CLHEP::Hep3Vector::y
double y() const
G4VSolid::kCarTolerance
G4double kCarTolerance
Definition: G4VSolid.hh:307
G4double
double G4double
Definition: G4Types.hh:76

Here is the call graph for this function:

Here is the caller graph for this function:

G4Trd & G4Trd::operator= ( const G4Trd &  rhs)

Definition at line 150 of file G4Trd.cc.

151 {
152  // Check assignment to self
153  //
154  if (this == &rhs) { return *this; }
155 
156  // Copy base class data
157  //
158  G4CSGSolid::operator=(rhs);
159 
160  // Copy data
161  //
162  fDx1 = rhs.fDx1; fDx2 = rhs.fDx2;
163  fDy1 = rhs.fDy1; fDy2 = rhs.fDy2;
164  fDz = rhs.fDz;
165 
166  return *this;
167 }
G4CSGSolid::operator=
G4CSGSolid & operator=(const G4CSGSolid &rhs)
Definition: G4CSGSolid.cc:91

Here is the call graph for this function:

void G4Trd::SetAllParameters ( G4double  pdx1,
G4double  pdx2,
G4double  pdy1,
G4double  pdy2,
G4double  pdz 
)

Definition at line 173 of file G4Trd.cc.

175 {
176  CheckAndSetAllParameters (pdx1, pdx2, pdy1, pdy2, pdz);
177 }
G4Trd::CheckAndSetAllParameters
void CheckAndSetAllParameters(G4double pdx1, G4double pdx2, G4double pdy1, G4double pdy2, G4double pdz)
Definition: G4Trd.cc:77

Here is the call graph for this function:

Here is the caller graph for this function:

void G4Trd::SetXHalfLength1 ( G4double  val)
inline
void G4Trd::SetXHalfLength2 ( G4double  val)
inline
void G4Trd::SetYHalfLength1 ( G4double  val)
inline
void G4Trd::SetYHalfLength2 ( G4double  val)
inline
void G4Trd::SetZHalfLength ( G4double  val)
inline
std::ostream & G4Trd::StreamInfo ( std::ostream &  os) const
virtual

Reimplemented from G4CSGSolid.

Definition at line 1238 of file G4Trd.cc.

1239 {
1240  G4int oldprc = os.precision(16);
1241  os << "-----------------------------------------------------------\n"
1242  << " *** Dump for solid - " << GetName() << " ***\n"
1243  << " ===================================================\n"
1244  << " Solid type: G4Trd\n"
1245  << " Parameters: \n"
1246  << " half length X, surface -dZ: " << fDx1/mm << " mm \n"
1247  << " half length X, surface +dZ: " << fDx2/mm << " mm \n"
1248  << " half length Y, surface -dZ: " << fDy1/mm << " mm \n"
1249  << " half length Y, surface +dZ: " << fDy2/mm << " mm \n"
1250  << " half length Z : " << fDz/mm << " mm \n"
1251  << "-----------------------------------------------------------\n";
1252  os.precision(oldprc);
1253 
1254  return os;
1255 }
G4VSolid::GetName
G4String GetName() const
mm
static constexpr double mm
Definition: G4SIunits.hh:115
G4int
int G4int
Definition: G4Types.hh:78

Here is the call graph for this function:

G4ThreeVector G4Trd::SurfaceNormal ( const G4ThreeVector &  p) const
virtual

Implements G4VSolid.

Definition at line 341 of file G4Trd.cc.

342 {
343  G4ThreeVector norm, sumnorm(0.,0.,0.);
344  G4int noSurfaces = 0;
345  G4double z = 2.0*fDz, tanx, secx, newpx, widx;
346  G4double tany, secy, newpy, widy;
347  G4double distx, disty, distz, fcos;
348  G4double delta = 0.5*kCarTolerance;
349 
350  tanx = (fDx2 - fDx1)/z;
351  secx = std::sqrt(1.0+tanx*tanx);
352  newpx = std::fabs(p.x())-p.z()*tanx;
353  widx = fDx2 - fDz*tanx;
354 
355  tany = (fDy2 - fDy1)/z;
356  secy = std::sqrt(1.0+tany*tany);
357  newpy = std::fabs(p.y())-p.z()*tany;
358  widy = fDy2 - fDz*tany;
359 
360  distx = std::fabs(newpx-widx)/secx; // perp. distance to x side
361  disty = std::fabs(newpy-widy)/secy; // to y side
362  distz = std::fabs(std::fabs(p.z())-fDz); // to z side
363 
364  fcos = 1.0/secx;
365  G4ThreeVector nX = G4ThreeVector( fcos,0,-tanx*fcos);
366  G4ThreeVector nmX = G4ThreeVector(-fcos,0,-tanx*fcos);
367 
368  fcos = 1.0/secy;
369  G4ThreeVector nY = G4ThreeVector(0, fcos,-tany*fcos);
370  G4ThreeVector nmY = G4ThreeVector(0,-fcos,-tany*fcos);
371  G4ThreeVector nZ = G4ThreeVector( 0, 0, 1.0);
372 
373  if (distx <= delta)
374  {
375  noSurfaces ++;
376  if ( p.x() >= 0.) sumnorm += nX;
377  else sumnorm += nmX;
378  }
379  if (disty <= delta)
380  {
381  noSurfaces ++;
382  if ( p.y() >= 0.) sumnorm += nY;
383  else sumnorm += nmY;
384  }
385  if (distz <= delta)
386  {
387  noSurfaces ++;
388  if ( p.z() >= 0.) sumnorm += nZ;
389  else sumnorm -= nZ;
390  }
391  if ( noSurfaces == 0 )
392  {
393 #ifdef G4CSGDEBUG
394  G4Exception("G4Trd::SurfaceNormal(p)", "GeomSolids1002", JustWarning,
395  "Point p is not on surface !?" );
396 #endif
397  norm = ApproxSurfaceNormal(p);
398  }
399  else if ( noSurfaces == 1 ) norm = sumnorm;
400  else norm = sumnorm.unit();
401  return norm;
402 }
G4ThreeVector
CLHEP::Hep3Vector G4ThreeVector
Definition: G4ThreeVector.hh:42
CLHEP::Hep3Vector::x
double x() const
fcos
G4double fcos(G4double arg)
Definition: G4tgrEvaluator.cc:68
JustWarning
Definition: G4ExceptionSeverity.hh:64
G4int
int G4int
Definition: G4Types.hh:78
CLHEP::Hep3Vector::z
double z() const
G4Trd::ApproxSurfaceNormal
G4ThreeVector ApproxSurfaceNormal(const G4ThreeVector &p) const
Definition: G4Trd.cc:410
G4Exception
void G4Exception(const char *originOfException, const char *exceptionCode, G4ExceptionSeverity severity, const char *comments)
Definition: G4Exception.cc:41
CLHEP::Hep3Vector::unit
Hep3Vector unit() const
CLHEP::Hep3Vector::y
double y() const
G4VSolid::kCarTolerance
G4double kCarTolerance
Definition: G4VSolid.hh:307
G4double
double G4double
Definition: G4Types.hh:76

Here is the call graph for this function:

The documentation for this class was generated from the following files: